Moving Charges And Magnetism

Magnetic field due to current element, Biot-Savart; Magnetic field on the axis of a circular current loop

• Static charges produce an electric field while current or moving charges produce magnetic field (B).

• Magnetic field of several sources is the vector addition of magnetic field of each individual source.

Lorentz Force

• Consider a point charge q moving in the presence of both electric and magnetic fields.

Let

q − Point charge

v − Velocity of point charge

t − Time

r − Distance

B (r) − Magnetic field

E (r) − Electric field

∴ Force on the charge, = F_electric + F_magnetic

This force is called Lorentz force.

• Force due to magnetic field depends on q, v, B. Force on negative charge is opposite to that of positive charge.

• Magnetic force is a vector product of velocity (v) and magnetic field (B). It vanishes, if v and B are parallel or anti-parallel.

• Magnetic force is zero, if charge is not moving.

• Unit of magnetic field (B) is tesla (T).

Magnetic Force on a Current Carrying Conductor Placed in Magnetic Field:

A straight rod carrying current is considered.

Let

A − Cross-sectional area of the rod

l − Length of the rod

n − Number density of mobile charge carriers

I − Current in the rod

v_d − Average drift velocity of mobile charge carrier

B − External magnetic field

Force on the carriers,

F = (nAl) qv_d × B

Since current density, j = nqv_d

∴ F = [(nqv_d)Al] × B

F = [jAl] × B

F = I l × B

Where,

l is the vector magnitude of length of the rod

• For a wire of arbitrary shape,

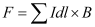
• Static charges produce an electric field while current or moving charges produce magnetic field (B).

• Magnetic field of several sources is the vector addition of magnetic field of each individual source.

Lorentz Force

• Consider a point charge q moving in the presence of both electric and magnetic fields.

Let

q − Point charge

v − Velocity of point charge

t − Time

r − Distance

B (r) − Magnetic field

E (r) − Electric field

∴ Force on the charge, = F_electric + F_magnetic

This force is called Lorentz force.

• Force due to magnetic field depends on q, v, B. Force on negative charge is opposite to that of positive charge.

• Magnetic force is a vector product of velocity (v) and magnetic field (B). It vanishes, if v and B are parallel or anti-parallel.

• Magnetic force is zero, if charge is not moving.

• Unit of magnetic field (B) is tesla (T).

Magnetic Force on a Current Carrying Conductor Placed in Magnetic Field:

A straight rod carrying current is considered.

Let

A − Cross-sectional area of the rod

l − Length of the rod

n − Number density of mobile charge carriers

I − Current in the rod

v_d − Average drift velocity of mobile charge carrier

B − External magnetic field

Force on the carriers,

F = (nAl) qv_d × B

Since current density, j = nqv_d

∴ F = [(nqv_d)Al] × B

F = [jAl] × B

F = I l × B

Where,

l is the vector magnitude of length of the rod

• For a wire of arbitrary shape,

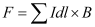
• When a charged particle having charge q moves inside a magnetic field 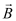with velocity v, it experiences a force .

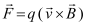• When 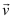is perpendicular to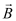, the force 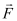on the charged particle acts as the centripetal force and makes it move along a circular path.

• Let m be the mass of charged particle and r be the radius of the circular path.

           Then $q\left(\overrightarrow{v}\times \overrightarrow{B}\right)=\frac{m{v}^2}{r}$

        v and B are at right angles

      $$\begin{gathered} \therefore qvB=\frac{m{v}^2}{r} \\ \Rightarrow r=\frac{mv}{Bq} \end{gathered}$$

• Time period of the circular motion of a charged particle is given by

            

        ⇒  

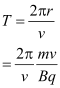
• Angular frequency,

         

This is often called cyclotron frequency.

Velocity Selector

• Force in the presence of magnetic and electric field,

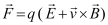
• Consider that the electric and the magnetic field are perpendicular to each other and, also, perpendicular to the velocity of the particle.
  Then we have:

       

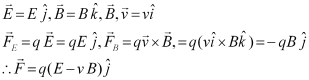
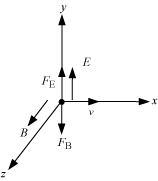
• If we adjust the values of 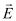and 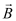such that magnitudes of the two forces are equal, then the total force on the charge will be zero and it will move in the fields undeflected. This happens when
  qE = qvB

        

• The above condition can be used to select a charged particle of particular velocity from the charges moving with different speeds. Therefore, it is called velocity selector.

Cyclotron

• It is used to accelerate charged particles or ions to …